what angle (in radians) corresponds to 0.75 rotations around the unit circle?

\(\require{abolish}\newcommand\degree[0]{^{\circ}} \newcommand\Ccancel[ii][blackness]{\renewcommand\CancelColor{\color{#1}}\cancel{#2}} \newcommand{\alert}[1]{\boldsymbol{\color{magenta}{#1}}} \newcommand{\blert}[1]{\boldsymbol{\color{bluish}{#1}}} \newcommand{\bluetext}[1]{\color{blueish}{#1}} \delimitershortfall-1sp \newcommand\abs[1]{\left|#1\right|} \newcommand{\lt}{<} \newcommand{\gt}{>} \newcommand{\amp}{&} \)

In the previous section, nosotros introduced periodic functions and demonstrated how they tin can be used to model real life phenomena like the rotation of the London Eye. In fact, there is an intuitive connection between periodic functions and the rotation of a circumvolve. In this department, we will utilise our intuition and formalize this connexion by exploring the unit circle and its unique features that lead us into the rich world of trigonometry.

Many applications involving circles also involve a rotation of the circle so we must first innovate a measure for the rotation, or angle, betwixt 2 rays (line segments) emanating from the middle of a circle.

The measure of an bending is a measurement between two intersecting lines, line segments, or rays, starting at the initial side and ending at the terminal side. It is a rotational measure, not a linear measure.

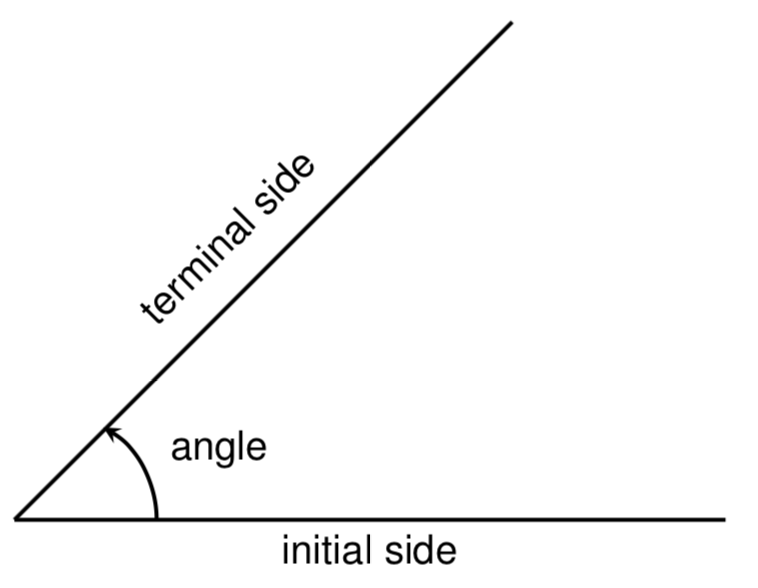
When measuring angles on a circle, unless otherwise directed, nosotros measure out angles in standard position: starting at the positive horizontal axis with a counterclockwise rotation.

Subsection Measuring Angles in Degrees

A degree is a unit of measurement of an bending. One rotation effectually a circle is equal to 360 degrees.

An angle measured in degrees should ever include the degree symbol \(^\circ\) or the word "degrees" later on the number. For example, \(90^\circ=ninety\) degrees.

Example 6

Give the degree measure of the angle shown on the circle.

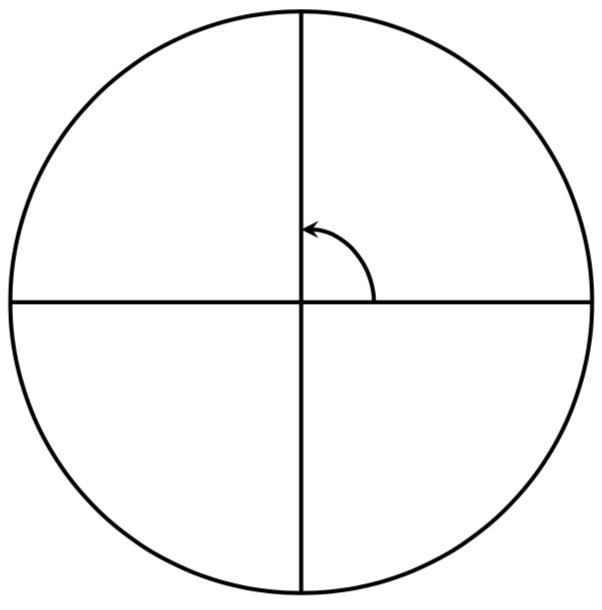
Solution

The vertical and horizontal lines separate the circle into quarters. Since one full rotation is 360 degrees, each quarter rotation is \(360^\circ / 4 = ninety^\circ\text{.}\)

Example vii

Describe an bending of \(thirty^\circ\) on a circle.

Solution

An angle of \(xxx^\circ\) is \(i/3\) of \(90^\circ\) then by dividing a quarter rotation into thirds, we can draw a line showing an angle of \(30^\circ\text{.}\)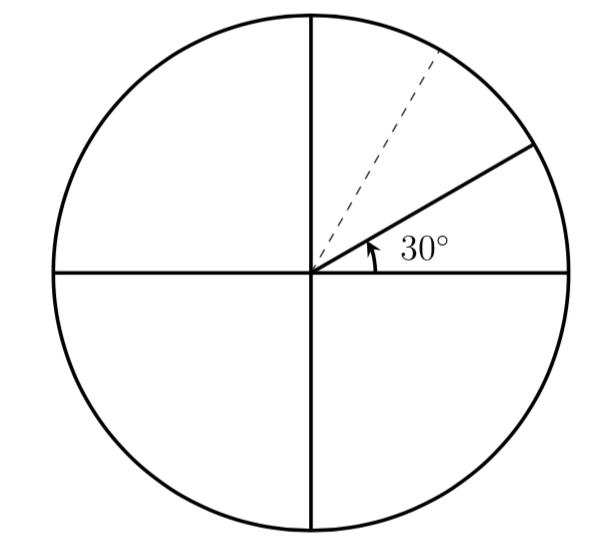

Notice that since there are 360 degrees in one rotation, an angle greater than 360 degrees would indicate more one full rotation. Shown on a circle, the resulting direction in which this angle'due south terminal side points is the same as another for an bending between 0 and 360. These angles are chosen coterminal.

After completing their full rotation based on the given angle, 2 angles are coterminal if they terminate in the same position, so their concluding sides coincide (point in the same direction).

Example 9

Find an angle \(\theta\) that is coterminal with \(800^\circ\) where \(0^\circ \leq \theta \lt 360^\circ \text{.}\)

Solution

Since adding or subtracting a full rotation, or 360 degrees, would result in an angle with the terminal side pointing in the same direction, we tin find coterminal angles by calculation or subtracting 360 degrees.

An angle of 800 degrees is coterminal with an angle of

\brainstorm{equation*} 800^\circ - 360^\circ = 440^\circ \cease{equation*}

It is also coterminal with an angle of

\begin{equation*} 440^\circ - 360^\circ = 80^\circ\text{.} \end{equation*}

Finding the coterminal bending between 0 and \(360^\circ\) can arrive easier to see which direction the concluding side of an angle points in.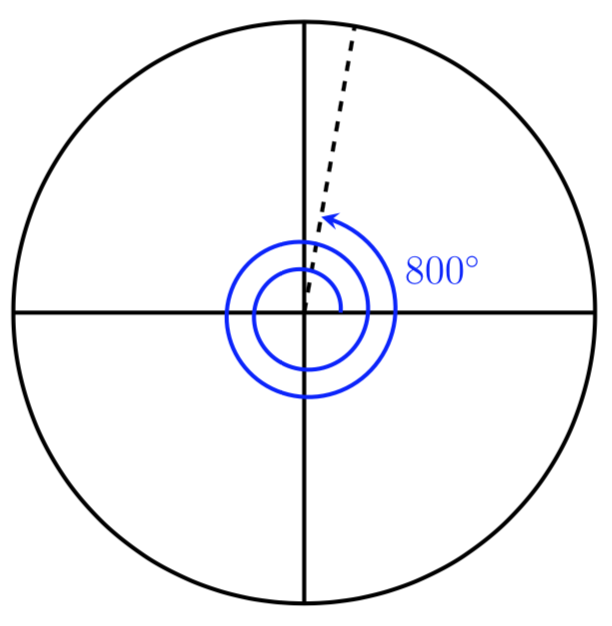

Example 10

Find an angle \(\alpha\) that is coterminal with \(870^\circ\) where \(0^\circ \leq \alpha \lt 360^\circ \text{.}\)

Solution

To observe angles with the final sides pointing in the same direction, we can subtract 360 degrees:

\brainstorm{equation*} \brainstorm{aligned} 870^\circ-360^\circ \amp= 510^\circ \\ 510^\circ-360^\circ \amp= 150^\circ \cease{aligned} \end{equation*}

Therefore \(870\) degrees is coterminal with \(150\) degrees.

Example 12

Depict an angle of \(-45^\circ\) on a circumvolve and find a positive coterminal angle \(\alpha\) where \(0^\circ \leq \alpha \lt 360^\circ \text{.}\)

Solution

Since 45 degrees is one-half of 90 degrees, we tin start at the positive horizontal axis and measure clockwise half of a ninety degree angle.

Nosotros tin notice a positive coterminal angle by adding 360 degrees.

\brainstorm{equation*} -45^\circ+360^\circ=315^\circ \cease{equation*}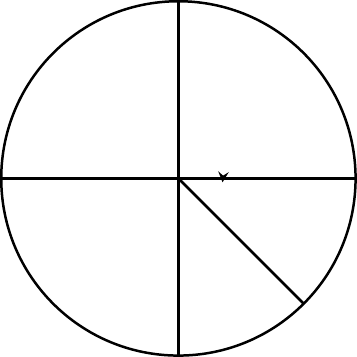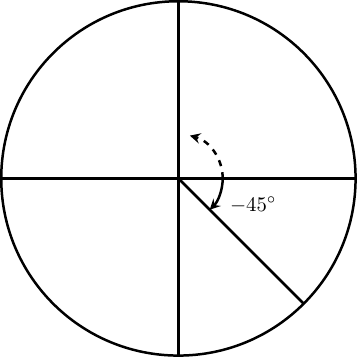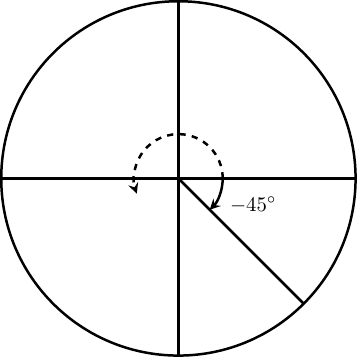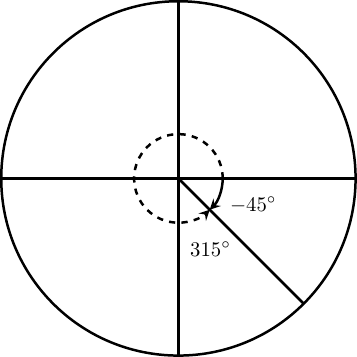

Example 13

Observe an angle \(\beta\) that is coterminal with \(-300^\circ\) where \(0^\circ \leq \beta \lt 360^\circ \text{.}\)

Solution

Since \(-300^\circ+360^\circ=60^\circ\text{,}\) \(60\) degrees is coterminal with \(-300^\circ\text{.}\)

Subsection Measuring Angles in Radians

While measuring angles in degrees may be familiar, doing so often complicates matters since the units of measure can go far the way of calculuations. For this reason, some other measure of angles is commonly used. This measure out is based on the altitude around the unit circumvolve.

The Unit Circumvolve

The unit of measurement circle is a circumvolve of radius i, centered at the origin of the \((x,y)\) plane. When measuring an angle around the unit circle, we travel in the counterclockwise direction, starting from the positive \(x\)-axis. A negative angle is measured in the opposite, or clockwise, direction. A complete trip effectually the unit of measurement circle amounts to a total of 360 degrees.

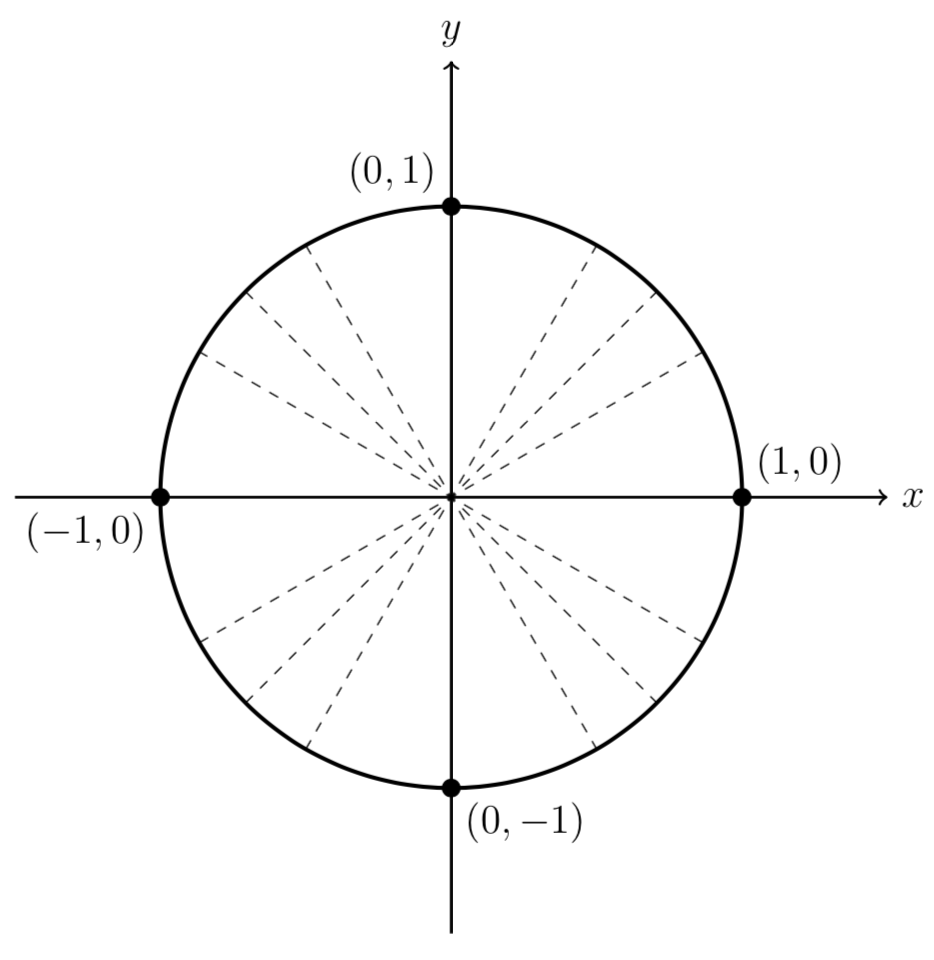
A radian is a measurement of an bending that arises from looking at angles every bit a fraction of the circumference of the unit circumvolve. A consummate trip effectually the unit circumvolve amounts to a total of \(2\pi\) radians.

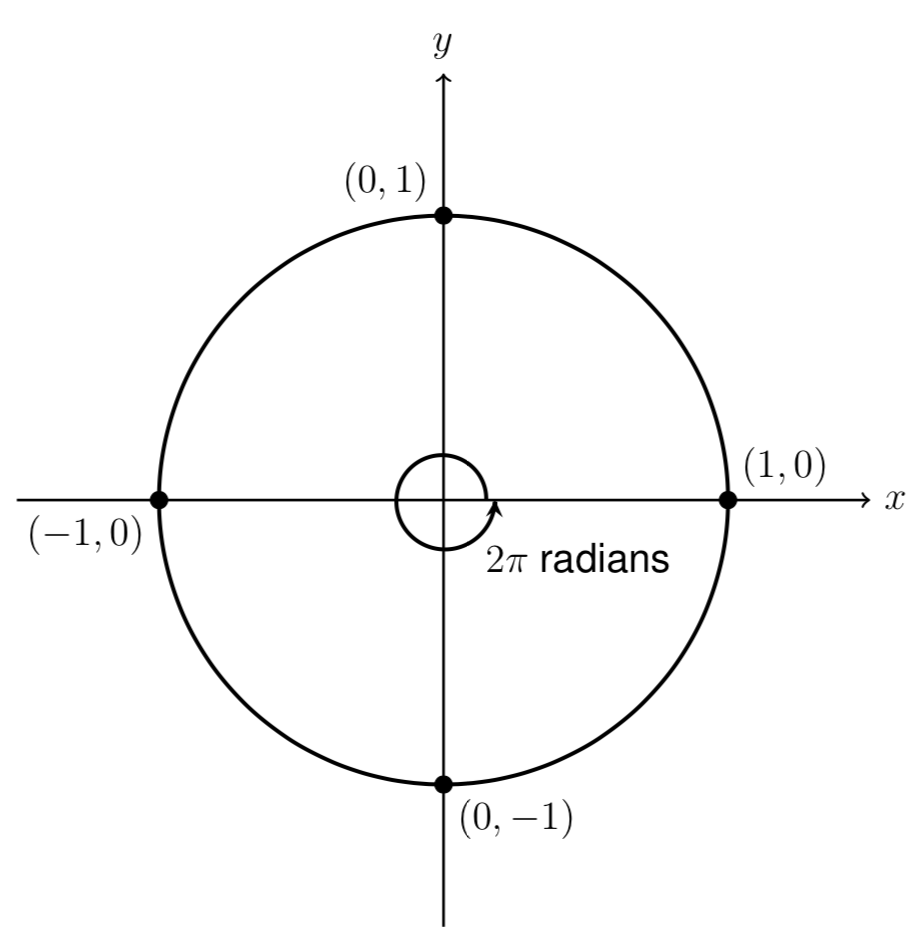
Radians are a unitless measure. Therefore, it is not necessary to write the characterization "radians" after a radian measure, and if you run across an bending that is not labeled with "degrees" or the degree symbol, you should assume that information technology is a radian measure.

Radians and degrees both measure angles. Thus, it is possible to convert between the two. Since one rotation around the unit circle equals 360 degrees or \(2\pi\) radians, nosotros tin use this as a conversion factor.

Converting Between Radians and Degrees

Since \(360 \text{ degrees} = 2\pi \text{ radians}\text{,}\) we can separate each side past 360 and conclude that

\begin{equation*} \displaystyle 1 \text{ degree} = \frac{2\pi \text{ radians}}{360} = \frac{\pi \text{ radians}}{180} \finish{equation*}

And so, to convert from degrees to radians, we tin can multiply by \(\displaystyle \ \frac{\pi \text{ radians}}{180^\circ}\)

Similarly, we can conclude that

\begin{equation*} \displaystyle 1 \text{ radian} = \frac{360^\circ}{two\pi} = \frac{180^\circ}{\pi} \terminate{equation*}

So, to convert from radians to degrees, we can multiply past \(\displaystyle \ \frac{180^\circ}{\pi \text{ radians}}\)

Example 15

Catechumen \(\displaystyle \frac{\pi}{6}\) radians to degrees.

Solution

Since we are given an angle in radians and we want to convert it to degrees, we multiply the angle past \(180^\circ\) and so carve up by \(\pi\) radians.

\begin{equation*} \frac{\pi}{half-dozen} \text{ radians} \cdot \frac{180^\circ}{\pi \text{ radians}} = 30^\circ \terminate{equation*}

Instance sixteen

Convert \(xv^\circ\) to radians.

Solution

In this case, nosotros get-go with an angle in degrees and desire to catechumen information technology to radians. Nosotros multiply by \(\pi\) and dissever by \(180^\circ\) and so that the units of degrees abolish and we are left with the unitless measure of radians.

\begin{equation*} 15^\circ \cdot \frac{\pi}{180^\circ} = \frac{\pi}{12} \finish{equation*}

Example 17

Convert \(\displaystyle \frac{seven\pi}{x}\) radians to degrees.

Solution

Since we are given an angle in radians and nosotros desire to convert it to degrees, we multiply by the angle \(180^\circ\) and then split by \(\pi\) radians.

\brainstorm{equation*} \frac{7\pi}{ten} \text{ radians} \cdot \frac{180^\circ}{\pi \text{ radians}} = 126^\circ \cease{equation*}

Above, we explored how to notice coterminal angles for angles greater than 360 degrees and less than 0 degrees. Similarly, we tin find coterminal angles for angles greater than \(2\pi\) radians and less than 0 radians.

Case xix

Find an bending \(\beta\) that is coterminal with \(\displaystyle \frac{11\pi}{4}\) where \(0^\circ \leq \beta \lt 2\pi \text{.}\)

Solution

When working in degrees, we institute coterminal angles by calculation or subtracting 360 degrees, a full rotation. Also, in radians, nosotros can find coterminal angles past adding or subtracting full rotations of \(2\pi\) radians.

An bending of \(11\pi/four\) is coterminal with an bending of

\brainstorm{equation*} \frac{eleven\pi}{4} - 2\pi = \frac{xi\pi}{four} - \frac{8\pi}{4} = \frac{3\pi}{4} \cease{equation*}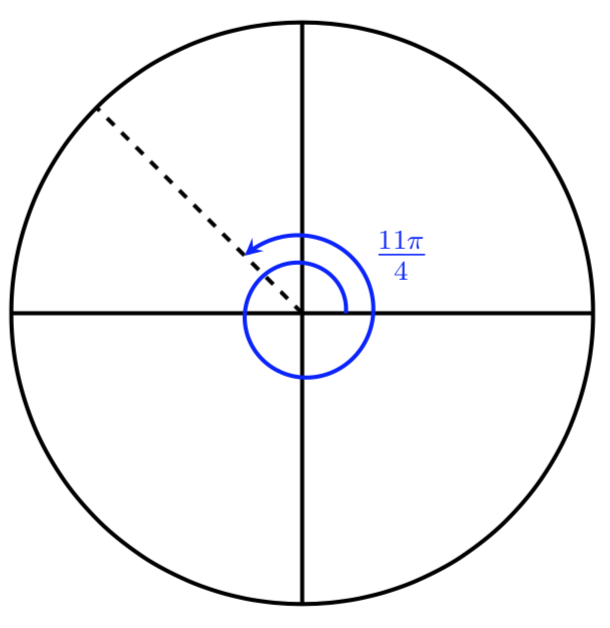

Example 20

Find an angle \(\phi\) that is coterminal with \(\displaystyle -\frac{11\pi}{half dozen}\) where \(0^\circ \leq \phi \lt 2\pi \text{.}\)

Solution

An angle of \(-\frac{11\pi}{vi}\) is coterminal with an angle of

\begin{equation*} -\frac{xi\pi}{6} + 2\pi = -\frac{11\pi}{6} + \frac{12\pi}{half dozen} = \frac{\pi}{half dozen} \end{equation*}

Subsection Finding Points on the Unit Circle

While it is user-friendly to describe the location of a point on the unit circle using an angle, relating this angle to the \(x\) and \(y\) coordinates of the corresponding point is an important application of trigonometry. To do this, nosotros volition demand to utilize our cognition of triangles.

Example 21

Discover the \((x,y)\) coordinates for the point on the unit of measurement circle corresponding to an angle of 45 degrees or \(\pi/4\) radians.

Solution

Let's starting time by drawing a picture and labeling the known information.

We desire to find the \(x\) and \(y\) coordinates of the indicate on the unit of measurement circle corresponding to an angle of 45 degrees or \(\pi/4\text{.}\) To exercise this, nosotros tin draw a vertical line from the point downwards to the \(10\)-axis, which forms a right triangle. The hypotenuse of this triangle is 1, since it corresponds to the radius of the unit circle, and the side lengths of this triangle are equal to \(x\) and \(y\text{.}\)

Now, using the Pythagorean Theorem, we go that

\begin{equation*} 10^ii+y^2=1^two \hspace{.25in} \text{ which simplifies to } \hspace{.25in} x^2+y^ii=1 \end{equation*}

Since the triangle formed is a 45-45-90 degree triangle, side lengths \(x\) and \(y\) must exist equal. Therefore, we can substitute in \(ten=y\) into the above equation.

\begin{marshal*} x^2+y^ii \amp = i \amp\amp \text{Substitute in } x=y \\ \\ 10^2+x^2 \amp = 1 \amp\amp \text{Add similar terms } \\ \\ 2x^2 \amp = ane \amp\amp \text{Divide by 2} \\ \\ x^2 \amp = \frac{i}{ii} \amp\amp \text{Take the square root} \\ \\ x \amp = \pm\sqrt{\vphantom{\frac{1^2}{2}}\frac{ane}{2}} \amp\amp \text{Since the } 10 \text{ value is positive, we continue the positive root so} \\ \\ x \amp = \sqrt{\vphantom{\frac{i^2}{ii}}\frac{1}{two}} \amp\amp \stop{marshal*}

Often this value is written with a rationalized denominator. Remember that to rationalize the denominator, we multiply past a term equivalent to one to get rid of the radical in the denominator, then

\brainstorm{equation*} x = \sqrt{\vphantom{\frac{one^2}{ii}}\frac{1}{ii}} \, \sqrt{\vphantom{\frac{i^2}{ii}}\frac{two}{2}} = \sqrt{\vphantom{\frac{1^2}{2}}\frac{ii}{4}} = \frac{\sqrt{2}}{two} \terminate{equation*}

and since \(x\) and \(y\) are equal, \(\displaystyle y=\frac{\sqrt{2}}{2}\text{.}\)

Thus, the \((ten,y)\) coordinates for the point on the unit circle corresponding to an angle of 45 degrees or \(\pi/four\) radians are

\brainstorm{equation*} (x,y) = \left(\frac{\sqrt{2}}{2},\frac{\sqrt{ii}}{ii}\right)\text{.} \end{equation*}

Example 22

Discover the \((x,y)\) coordinates for the point on the unit of measurement circle corresponding to an angle of thirty degrees or \(\pi/six\) radians.

Solution

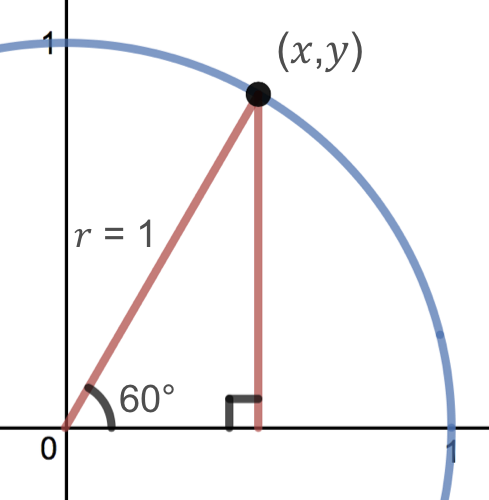
Permit'south start by drawing a flick and labeling the known information.

We want to find the \(ten\) and \(y\) coordinates of the betoken on the unit of measurement circle corresponding to an angle of thirty degrees or \(\pi/6\text{.}\) To do this, we can draw a triangle inside the unit circle with 1 side at an angle of xxx degrees and another at an angle of \(-xxx\) degrees.

Notice that if we combine the resulting two correct triangles to form i large triangle, then all three angles of the larger triangle are equal to lx degrees.

Since all of the angles in this triangle are equal, the sides will all be equal besides. Ii of the side lengths of this triangle are equal to 1 because they correspond to the radius of the unit circle. Thus, the other side length must also exist equal to 1. This side also has a length of \(2y\text{.}\) Therefore, nosotros can conclude that \(2y = one\) and then

\begin{equation*} y=\frac{1}{two} \stop{equation*}

Now, we tin utilise the Pythagorean Theorem to 1 of the right triangles to notice the \(x\) value. Nosotros get that

\begin{marshal*} x^2+y^ii \amp = 1^2 \\ \\ x^2+\left(\frac{one}{2}\right)^2 \amp = ane \\ \\ x^2+\frac{1}{iv} \amp = 1 \\ \\ x^2 \amp = \frac{3}{4} \\ \\ 10 \amp = \pm\sqrt{\vphantom{\frac{iii^2}{4}}\frac{3}{4}} \\ \\ x \amp = \sqrt{\vphantom{\frac{3^two}{four}}\frac{three}{4}} = \frac{\sqrt{3}}{2} \end{marshal*}

Thus, the \((x,y)\) coordinates for the signal on the unit circle corresponding to an angle of 30 degrees or \(\pi/6\) are

\begin{equation*} (x,y) = \left(\frac{\sqrt{3}}{2},\frac{one}{2}\right)\text{.} \finish{equation*}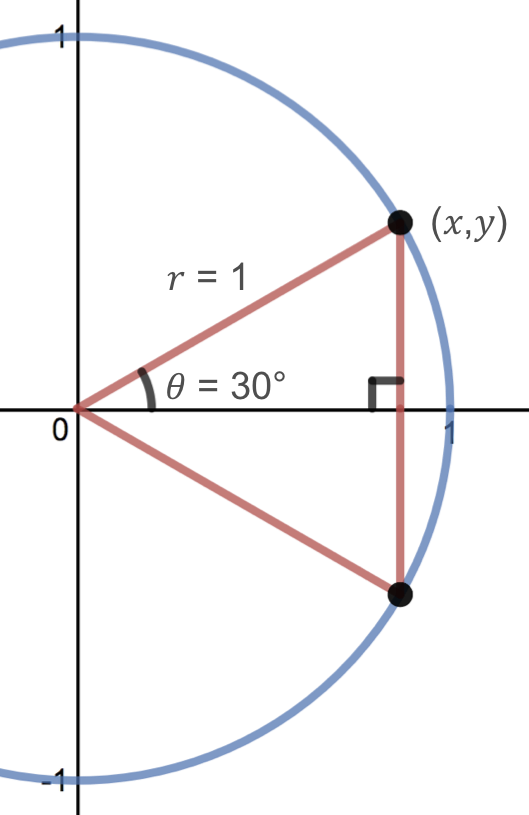

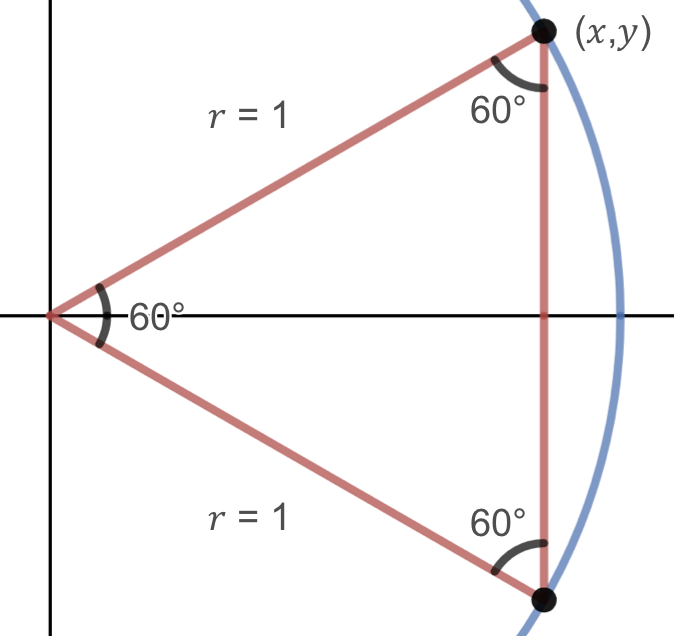
In the previous case, we applied our knowledge of triangles to find the \(x\) and \(y\) coordinates of the point on the unit circumvolve corresponding to 30 degrees. Nosotros can now apply symmetry to find the \(x\) and \(y\) coordinates respective to an angle of 60 degrees or \(\pi/three\text{.}\) Beginning, we draw a picture of the triangle corresponding to this betoken on the unit circle.

Notice that the triangle shown above is similar to the ane formed by the xxx degree angle since the hypotenuse is the aforementioned length and both are 30-60-90 caste triangles. Therefore, the \((ten,y)\) coordinates for sixty degrees are the same every bit the \((x,y)\) coordinates for 30 degrees, but switched.

Thus, the \((x,y)\) coordinates for the point on the unit circle corresponding to an angle of sixty degrees or \(\pi/3\) are

\brainstorm{equation*} (x,y) = \left(\frac{one}{2},\frac{\sqrt{3}}{2}\correct)\text{.} \end{equation*}

Nosotros have now constitute the coordinates of the points corresponding to all the ordinarily encountered angles in the first quadrant of the unit of measurement circle. Using symmetry, we can observe the balance of the coordinates respective to mutual angles on the unit circle. A labeled movie of the unit circle is shown beneath.

Subsection Supplemental Videos

• Measuring Angles
• Coordinates on the Unit of measurement Circle
• Examples

Subsection Exercises

1 Converting Degrees to Radians

2 Converting Radians to Degrees

iii Converting Between Degrees and Radians

iv Practical Converting Between Degrees and Radians

jessiehismay.blogspot.com

Source: https://mathbooks.unl.edu/PreCalculus/unit-circle.html

Share this post